Both Newton and Einstein claimed that gravitational acceleration is independent of the mass or the energy being accelerated. Another idea that Newton and Einstein professed, was the equivalency of inertial mass and gravitational mass. Newton assumed these two mass units were equal without giving a reason for thinking why they should be equal. This disturbed Einstein, he wanted to know the reason why they are equal. Newton also said in the Principia, "We have explained the phenomena of the heavens and of our sea by the power of gravity, but have not yet assigned the cause of this power... I have not been able to discover the cause of those properties of gravity from the phenomena, and I frame no hypotheses; for what ever is not deduced from phenomena is to be called an hypothesis; and hypothesis, whether metaphysical or physical, whether of occult qualities or mechanical, have no place in experimental philosophy." Mook 148
Einstein did not provide an answer to this question raised by Newton concerning the "cause" of gravity, but he did create a much more accurate model of gravity than did Newton; and, perhaps more significant, Einstein's model is far richer in its consequences. Nevertheless, one is still left with a question that asks: "How is it possible...?" Just as with Newton's model, which leads to action at a distance without a real cause, Einstein's model leaves one with a geometric curvature of space without a cause. Einstein worked till his dyeing day seeking that cause, as well as a theory that would unify the forces of nature, but to no avail. Einstein was not satisfied with Newton's simple acceptance of the principle of equivalence; he devised an idea that was to explain this principle. He claimed the following: -
"In any small region of space, the effects produced by gravitation are the same as those by an acceleration." 141
The following is a modern version of his idea. He devised a laboratory room in a spaceship, remote from any other mass influence, equipped with a rocket motor at the bottom, and the rocket is turned off. Now he turns on the rocket motor; one feels himself pressed down to the floor of the laboratory due to the constant acceleration. A ball thrown up in the room comes down with the same speed as the spaceship, for instance, 32 ft. per sec., per sec.
He now moves the rocket ship to the earth and simply hovers above the ground. Again, a ball thrown up in the room comes down, 32 ft per second, per second. Einstein thus proves, in any small region of space, the effect produced by acceleration is the same as the effect produced by gravity. It was a profound realization in the life of physics at that time; it proved that the inertial charge of mass was equal to the gravitational charge of mass. Nevertheless, no way did it explain what was inertial mass or a cause for the gravity charge. It just maintained that they are equal to one another, thus, providing an unaccountable insight.
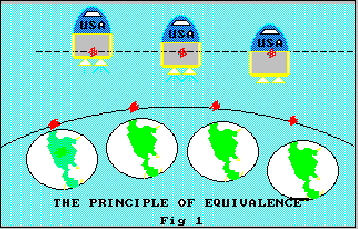
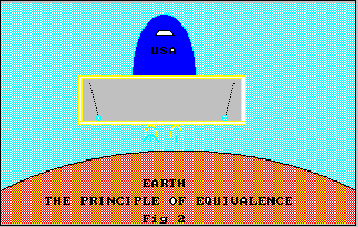
Einstein's principle of equivalence began with the qualification: "in any small region of space."
This is required for if a rocket ship laboratory room was as wide as the planet above which it
hovered, and a ball was released at both ends of the room at some height above the floor, then, due
to the force of gravity they would tend to come together as they descended. This is because they
would be heading toward the center of the planet, and certainly to the bottom of the room;
nevertheless, in an accelerated room the same size away from the planet, they would fall straight
down to the floor of the room at both ends. See Fig 1, Fig 2

Another principle that Einstein postulated in "The Foundation of the General Theory of Relativity" is a principle also found in the original special theory of relativity. "The laws of physics must be the same for all observers moving in inertial (non-accelerated) reference frames." 139
According to this principle there are no privileged inertial reference frames in the universe. In
the special theory this principal holds true, but Einstein wanted to extend this principle to a
general theory for all motions: -
"The laws of physics must be of such a nature that they apply to systems of reference in any kind of motion." 139
In the special theory Einstein did not include accelerated reference frames, because with an actual experiment, one can feel this acceleration of its own accord, and without reference to any outside source. In other words, accelerated systems appear to be special or privileged, their acceleration seemed to be absolute, which is something that Einstein did not like about Newton's theory of absolute space, absolute time, and absolute motion. Einstein sought to extend the notion of equality of perspective, established for non- accelerating reference frames in special relativity, by stating that any and all frames of reference are equally valid for expressing the laws of physics. Einstein realized he had a problem that had to be solved first before he could extend his special theory to a general theory. Einstein solved this problem with what he called ---"the happiest thought of my life;" a thought he developed into the principle of equivalence. Einstein said of his happy though: "I was sitting in a chair in the patent office at Bern when all of a sudden a thought occurred to me: 'If a person falls freely he will not feel his own weight,' I was startled. This simple thought made a deep impression on me. It impelled me toward a theory of gravitation." 141
For instance, standing on a scale inside an airplane, setting on the apron of the runway ready
to take off, a person gets on a scale and finds he weighs 150 lbs. The plane takes off and flies
50,000 feet into the sky. The pilot puts the plane into a nose dive, 32 ft. per second, per second;
standing on the scale again, he does not register any weigh at all. Why is he weightless? The pilot
pulls out of the dive; the person puts on a parachute and jumps out. He has that same feeling of
weightlessness he had in the plane when it was in a dive at 32 ft per second, per second. Newton says,
he better start worrying, the earth is reaching out grabbing a hold of him, dragging him back to earth,
so, he had better actuate his chute. Newton calculates the force by multiplying the universal
gravitational constant times the mass of the earth and the person's mass, and divides the product by
the square of the distance between the person and the earth. He claims the attractive force of gravity
has a hold of the individual, and it will bring him down to earth. Newton claims that the person is
reaching out grabbing a hold of the earth and pulling the earth toward him; this in accordance with
Newton's third law, but the person, of course, has little or no effect on the earth. F = G M m / r^2,
this is Newton's equation for the law of gravity.
Newton figured that a force is acting upon the falling individual; because the first law states: every
body continues in a state of rest, or of uniform motion in a straight line, unless compelled to change
that state by a force impressed upon it. In a person's free fall situation; Newton claims the force of
the earth's gravity is reaching out, pulling him down to the earth. Einstein knew he had his work
cut out for him. He had to eliminate that action at a distance; a notion which even Newton did not
like to admit, yet had to accept for the want of a better reason. Newton did not like the idea, and
said so to friends. Einstein thought about the notion of the free fall affect. He figured this same
affect would happen to an object floating around in a box if the box suddenly accelerated up at 32
ft per second, per second. The object then would appear to fall to the bottom of the box to any one
in the box at the rate of 32 ft per second, per second. Hence, its gravitational mass is equal to its
inertial mass; consequently, the effect of acceleration produces the effect of gravitation. Now all he
had to do was to accelerate a falling person to the earth without the earth acting upon the person with
the action at a distance which, at that time, did not make any sense to Einstein.